Inverse problems of diffractive optics synthesis

The first flat optical elements were proposed by Augustin Fresnel in the 18th century. Such elements, which were used to focus light to a point, were amplitude-based, and their energy losses exceeded 50%. The sweeping development of flat optics coincided with the emergence of lasers. The breakthrough results in this field were obtained in the second half of the past century. Flat optical elements now perform a wide range of tasks that involve shaping of laser radiation, and are used in laser monitoring, laser thermohardening, laser marking, etc.

The synthesis of flat optical elements consists of computing the microrelief of the optical element and then shaping this microrelief. Inverse problems of the synthesis of flat optical elements involve the use of various models: ray optics approximation, scalar wave model of Fresnel and Kirchhoff, and Maxwell's equations. In scalar wave model the wave function u(x,y,-0) of the radiation incident onto the optical element located in the Z=0 plane is related to the scalar wave function u(x,y,+0) of the radiation after the optical element by the following formula:

u(x,y,+0) = u(x,y,-0) e^ikφ(x,y).

Here k=2π/λ and φ(x,y) is the phase function of the flat optical element. In the Fresnel‘s model the problem of the synthesis of a flat optical element for shaping the given image or the given beam pattern reduces to solving the following nonlinear operator equation:

Aφ=| ∫∫ K(x,y,ξ,η) eikφ(ξ,η) dξ dη |=F(x,y) G

(1)

Here K(x,y,ξ,η), F(x,y) are the given functions, and G is the domain of the flat optical element in the Z=0 plane.

The task of the synthesis of flat optical elements is complicated by a number of problems. These include the fact that the inverse problem of the synthesis of flat optics is not always solvable. Even if a solution exists, it may be non-unique. The inverse problem is ill posed. Methods of solving such problems in terms of various mathematical models were detailed in the monograph [1]

Figure 1(a) shows the photo of a flat optical element made on a silicon plate and used in a powerful CO₂ industrial laser with a 35 mm beam diameter. The wavelength and microrelief depth are equal to 10.6μm and about 10 μm, respectively.

Over the past five years the most interesting results in flat computer optics were those obtained in problems of the shaping of optical radiation. Such elements, which have a microrelief with a depth on the order of 0.1 – 0.3 μm carved with an accuracy of 10 – 20 nm, are now referred to as "nanooptical". The microrelief of nanooptical elements is shaped using the technology of electron-beam lithography.

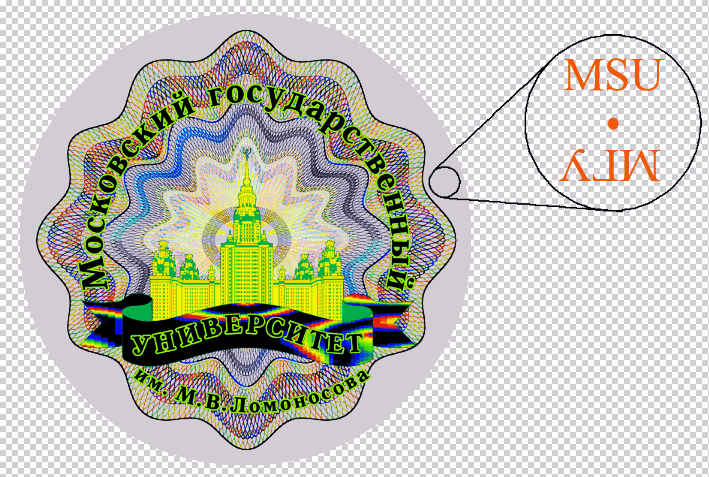
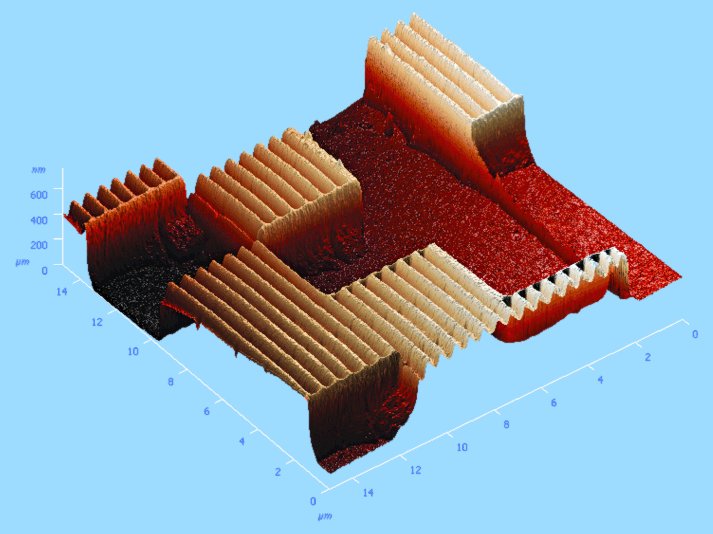
One of the most interesting applications of nanooptical elements are optical security technologies. Nanooptical elements offer a wide range of security features both for visual and expert control. Figure 1(b) shows a general view of an optical security element including visual security features and concealed security features used for expert control. The latter include the so-called CLR (Covert Laser Readable) images. The figure shows an example of a multigrade CLR image ("MSU") visualizable with laser radiation. The images in the -1 and +1 diffraction orders do not coincide. Such an image can be shaped only with asymmetric microrelief [2]. Figure 1(c) shows a fragment of the microrelief of a nanooptical security element scanned with an atomic force microscope. Counterfeit protection is guaranteed by the highly knowledge-intensive nature of the technology and high cost of the equipment used for E-beam lithography. These solutions, which were developed by our laboratory, are used to protect documents in the Russian Federation.